HDU-1828-Picture(线段树)

A number of rectangular posters, photographs and other pictures of the same shape are pasted on a wall. Their sides are all vertical or horizontal. Each rectangle can be partially or totally covered by the others. The length of the boundary of the union of all rectangles is called the perimeter.

Write a program to calculate the perimeter. An example with 7 rectangles is shown in Figure 1.



The corresponding boundary is the whole set of line segments drawn in Figure 2.



The vertices of all rectangles have integer coordinates.

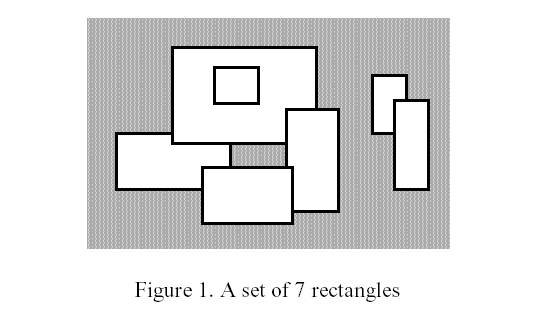
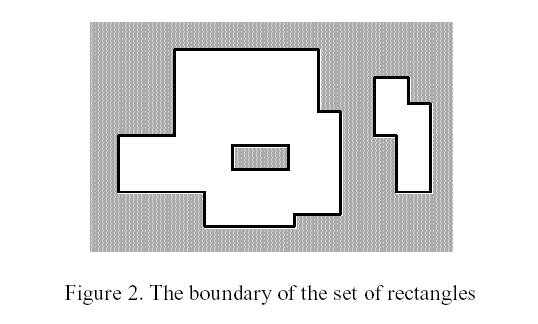
7
-15 0 5 10
-5 8 20 25
15 -4 24 14
0 -6 16 4
2 15 10 22
30 10 36 20
34 0 40 16

228

思路：求纵向边要维护重叠次数大于0的纵向长度，用上一次的node[1].m减去当前的node[1].m的绝对值。求横向边要维护每一次更新之后需要加上去的边数。

#include <cstdio>
#include <cmath>
#include <algorithm>
using namespace std;

struct L{
int val;//1 左边，-1 右边
int x,y1,y2;
}l[10000];

struct N{
int l,r,n,m;//n记录节点对应的有效横向边数量，m记录纵向长度
int cnt;//重叠情况
bool lc,rc;
}node[40000];

int tempy[10000];

bool cmp(struct L a,struct L b)
{
    if(a.x==b.x) return a.val>b.val;

    return a.x<b.x;
}

void build(int idx,int s,int e)
{
    node[idx].cnt=0;
    node[idx].m=0;
    node[idx].lc=node[idx].rc=0;
    node[idx].l=tempy[s-1];
    node[idx].r=tempy[e-1];

    if(s+1!=e)
    {
        int mid=(s+e)>>1;

        build(idx<<1,s,mid);
        build(idx<<1|1,mid,e);
    }
}

void len(int idx,int s,int e)
{
    if(node[idx].cnt>0)
    {
        node[idx].m=node[idx].r-node[idx].l;
        node[idx].n=2;//对应两条边
        node[idx].lc=node[idx].rc=1;
    }
    else
    {
        if(s+1!=e)
        {
            int mid=(s+e)>>1;

            node[idx].m=node[idx<<1].m+node[idx<<1|1].m;
            node[idx].n=node[idx<<1].n+node[idx<<1|1].n;
            node[idx].lc=node[idx<<1].lc;
            node[idx].rc=node[idx<<1|1].rc;
            if(node[idx<<1].rc && node[idx<<1|1].lc) node[idx].n-=2;//如果左右儿子是连着的，就要减去多计算的两条边
        }
        else
        {
            node[idx].m=0;
            node[idx].n=0;
            node[idx].lc=node[idx].rc=0;
        }
    }
}

void update(int idx,int s,int e,struct L line)
{
    if(node[idx].l==line.y1 && node[idx].r==line.y2)
    {
        node[idx].cnt+=line.val;
        len(idx,s,e);
        return;
    }

    if(s+1!=e)
    {
        int mid=(s+e)>>1;

        if(line.y2<=node[idx<<1].r) update(idx<<1,s,mid,line);
        else if(line.y1>=node[idx<<1|1].l) update(idx<<1|1,mid,e,line);
        else
        {
            int temp=line.y2;

            line.y2=node[idx<<1].r;
            update(idx<<1,s,mid,line);
            line.y2=temp;

            line.y1=node[idx<<1|1].l;
            update(idx<<1|1,mid,e,line);
        }

    }

    len(idx,s,e);
}

int main()
{
    int T,n,i,t;
    int x1,x2,y1,y2;

    while(~scanf("%d",&n))
    {
        for(i=0;i<n;i++)
        {
            scanf("%d%d%d%d",&x1,&y1,&x2,&y2);

            tempy[i*2]=y1;
            tempy[i*2+1]=y2;

            l[i*2].val=1;
            l[i*2].x=x1;
            l[i*2].y1=y1;
            l[i*2].y2=y2;

            l[i*2+1].val=-1;
            l[i*2+1].x=x2;
            l[i*2+1].y1=y1;
            l[i*2+1].y2=y2;
        }

        sort(tempy,tempy+n*2);
        sort(l,l+n*2,cmp);

        t=unique(tempy,tempy+n*2)-tempy;//去重

        build(1,1,t);

        int ans=0,last=0;

        for(i=0;i<n*2;i++)
        {
            if(i) ans+=node[1].n*(l[i].x-l[i-1].x);

            update(1,1,t,l[i]);

            ans+=abs(node[1].m-last);
            last=node[1].m;
        }

        printf("%d\n",ans);
    }
}

HDU-1828-Picture(线段树),布布扣,bubuko.com

HDU-1828-Picture(线段树)

原文：http://blog.csdn.net/faithdmc/article/details/37558639