题解[UVA839]天平 Not so Mobile

Description

Input

Output

Examples

Input

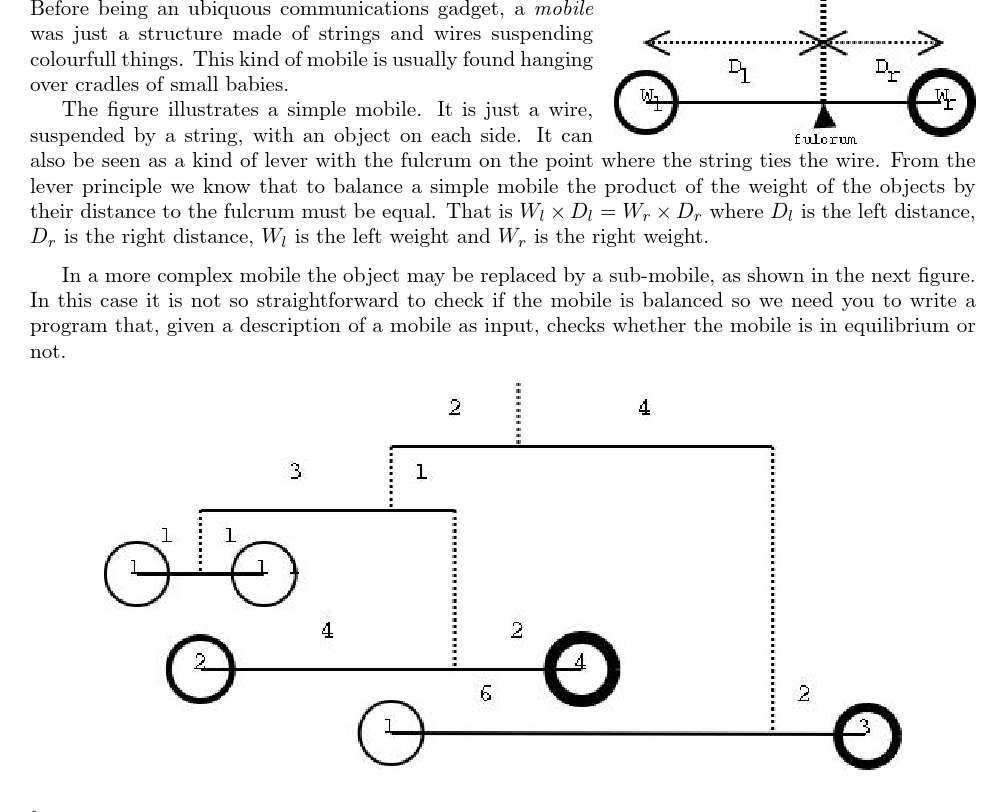
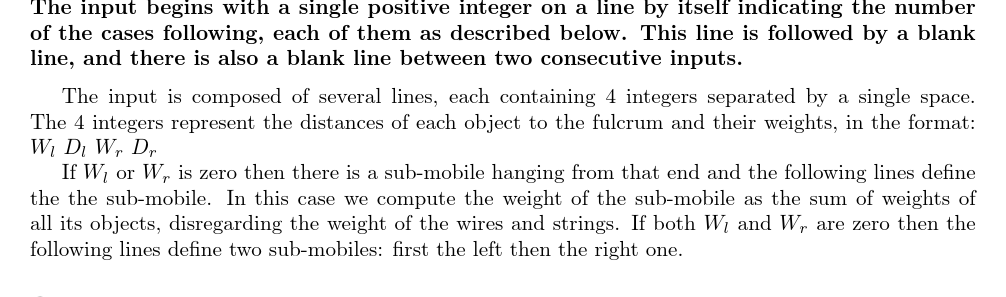
1
0 2 0 4
0 3 0 1
1 1 1 1
2 4 4 2
1 6 3 2

Output

YES

Translation

输入一个树状天平，根据力矩相等原则判断是否平衡。

采用递归（先序）方式输入：每个天平格式为\(W_l,D_l,W_r,D_r\)，当\(W_l\)或\(W_r\)为\(0\)时，表示该“砝码”实际是一个子天平，接下来会描述这个子天平。当\(W_l=W_r=0\)时，会先描述左子天平，然后是右子天平。

其正确输出为\(YES\)，否则输出\(NO\)。

Solution

这道题目的输入就采取了递归方式定义，所以编写一个递归函数进行输入比较自然。实际上，在输入的过程中就可以完成判断。由于使用了引用传值，因此，代码很精简。

本题极为重要，是递归的好题。

Code

#include <iostream>
#include <cstdio>
#include <cstdlib>
#include <cstring>
#include <algorithm>
#include <cmath>
#include <cctype>

using namespace std;

inline int gi()
{
    int f = 1, x = 0; char c = getchar();
    while (c < '0' || c > '9') { if (c == '-') f = -1; c = getchar();}
    while (c >= '0' && c <= '9') { x = x * 10 + c - '0'; c = getchar();}
    return f * x;
}

int t, y;//t为询问总数,y为子天平的总重量

bool pd(int &x)//输入一个子天平，返回子天平是否平衡，参数x为当前子天平的总重量
{
    int a, b, c, d;//输入顺序同题目翻译
    bool f = true, fl = true;//判断有没有子天平
    a = gi(), b = gi(), c = gi(), d = gi();//输入
    if (!a) f = pd(a);//左边有子天平
    if (!c) fl = pd(c);//右边有子天平
    x = a + c;//修改总重量
    return (f && fl && (a * b == c * d));//进行判断
}

int main()//进入主函数
{
    t = gi();//输入数据组数
    while (t--)
    {
        if (pd(y)) puts("YES");//如果合法就输出YES
        else puts("NO");//否则输出NO
        if (t) puts("");//每组数据用空行隔开，最后一组数据后不要输出空行
    }
    return 0;//结束主函数
}

题解[UVA839]天平 Not so Mobile

原文：https://www.cnblogs.com/xsl19/p/11104958.html