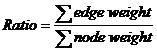
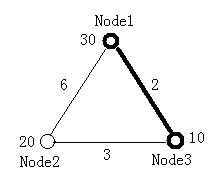
3 2 30 20 10 0 6 2 6 0 3 2 3 0 2 2 1 1 0 2 2 0 0 0
1 3 1 2
#include <cstdio>
#include <cstring>
#include <algorithm>
#define MAX 20
#define INF 100000000
using namespace std ;
int graph[MAX][MAX] , lowCost[MAX] , node[MAX] , path[MAX] , temp[MAX];
int n ,m ;
bool cmp(const int a , const int b)
{
return a<b ;
}
int prim(int s)
{
bool visited[MAX] ;
memset(visited,false,sizeof(visited)) ;
int sum = 0 ;
for(int i = 0 ; i < m ; ++i)
{
lowCost[temp[i]] = graph[s][temp[i]] ;
}
visited[s] = true ;
for(int i = 0 ; i < m-1 ; ++i)
{
int min = INF , index = -1 ;
for(int j = 0 ; j < m ; ++j)
{
if(!visited[temp[j]] && lowCost[temp[j]]<min)
{
min = lowCost[temp[j]] ;
index = temp[j] ;
}
}
if(index == -1)
{
break ;
}
visited[index] = true ;
sum += min ;
for(int j = 0 ; j < m ; ++j)
{
if(!visited[temp[j]] && lowCost[temp[j]]>graph[index][temp[j]])
{
lowCost[temp[j]] = graph[index][temp[j]] ;
}
}
}
return sum ;
}
double ans = INF*1.0 ;
void DFS(int num , int count)
{
if(count == m)
{
int sumOfEdge = prim(temp[0]) ,sumOfNode = 0;
for(int i = 0 ; i < m ; ++i)
{
sumOfNode += node[temp[i]] ;
}
double t = sumOfEdge*1.0/sumOfNode ;
if(ans-t>0.00001)
{
ans = t ;
for(int i = 0 ; i < m ; ++i)
{
path[i] = temp[i] ;
}
}
return ;
}
for(int i = num+1 ; i <= n ; ++i)
{
temp[count] = i ;
DFS(i,count+1);
}
}
int main()
{
while(~scanf("%d%d",&n,&m) && (m||n))
{
for(int i = 1 ; i <= n ; ++i)
{
scanf("%d",&node[i]) ;
}
for(int i = 1 ;i <= n ; ++i )
{
for(int j = 1 ; j <= n ; ++j)
{
scanf("%d",&graph[i][j]) ;
}
graph[i][i] = INF ;
}
ans = INF*1.0 ;
for(int i = 1 ; i <= n ; ++i)
{
temp[0] = i ;
DFS(i,1);
}
sort(path,path+m,cmp) ;
for(int i = 0 ; i < m ; ++i)
{
printf("%d",path[i]) ;
if(i != m-1)
{
printf(" ") ;
}
}
puts("") ;
}
return 0 ;
}hdu 2489 Minimal Ratio Tree DFS枚举点+最小生成树 属于中等偏上题 ,Double比较大小的时候注意精度问题
原文:http://blog.csdn.net/lionel_d/article/details/43919601